Why It’s Impossible to Know a Coastline’s True Length

Imagine, for a moment, that you and your friend have been given a seemingly straightforward task: to measure the coastline of Puget Sound, in Washington State. Resources are tight, so you’ve got a yardstick, while your friend has a foot-long ruler. You each walk along, laying your measuring stick along the edge of the water, following the the ins and outs of the shore as best you can. When you’re finished, you compare notes—and you’re shocked. While you ended up with a respectable 3,000 miles, your friend and his foot-long got a way higher number, somewhere around 4,500 miles.

You guys aren’t crazy. You’re victims of the coastline paradox, a tricky mathematical principle that messes with cartographers, stymies government bureaus, and makes it impossible to know exactly how big our world truly is.

People have been confused by coastlines since at least the fifth century B.C., when Athenian sailors were reportedly tasked with measuring the coast of Sardinia and came back baffled. But the paradox first rigorously revealed itself in 1951, during a study of armed conflict. Lewis Fry Richardson, a pacifist and mathematician, was trying to figure out whether the length of the border shared by two given countries had any bearing on whether or not they would go to war.

He saw disagreement even in the data-gathering phase—while seeking the length of the Spain-Portugal border, he found that Spain had it plotted as 987 km, while Portugal said 1214 km. Fascinated by this discovery, he looked into it further, and worked out that not only are the lengths of coastlines and certain borders extremely variable—if you get a small enough measuring stick, each is effectively infinitely long.

This line of thinking was soon picked up by mathematician Benoit Mandelbrot, in a 1967 paper called “How long is the coast of Britain?” “Here is a question, a staple of grade-school geometry that, if you think about it, is impossible,” he later told the New York Times. In order to tackle it—and to measure other similarly wonky shapes, like clouds, snowflakes and mountains—Mandelbrot invented the concept of the fractal, a curve that gets more complex the more closely you look at it.

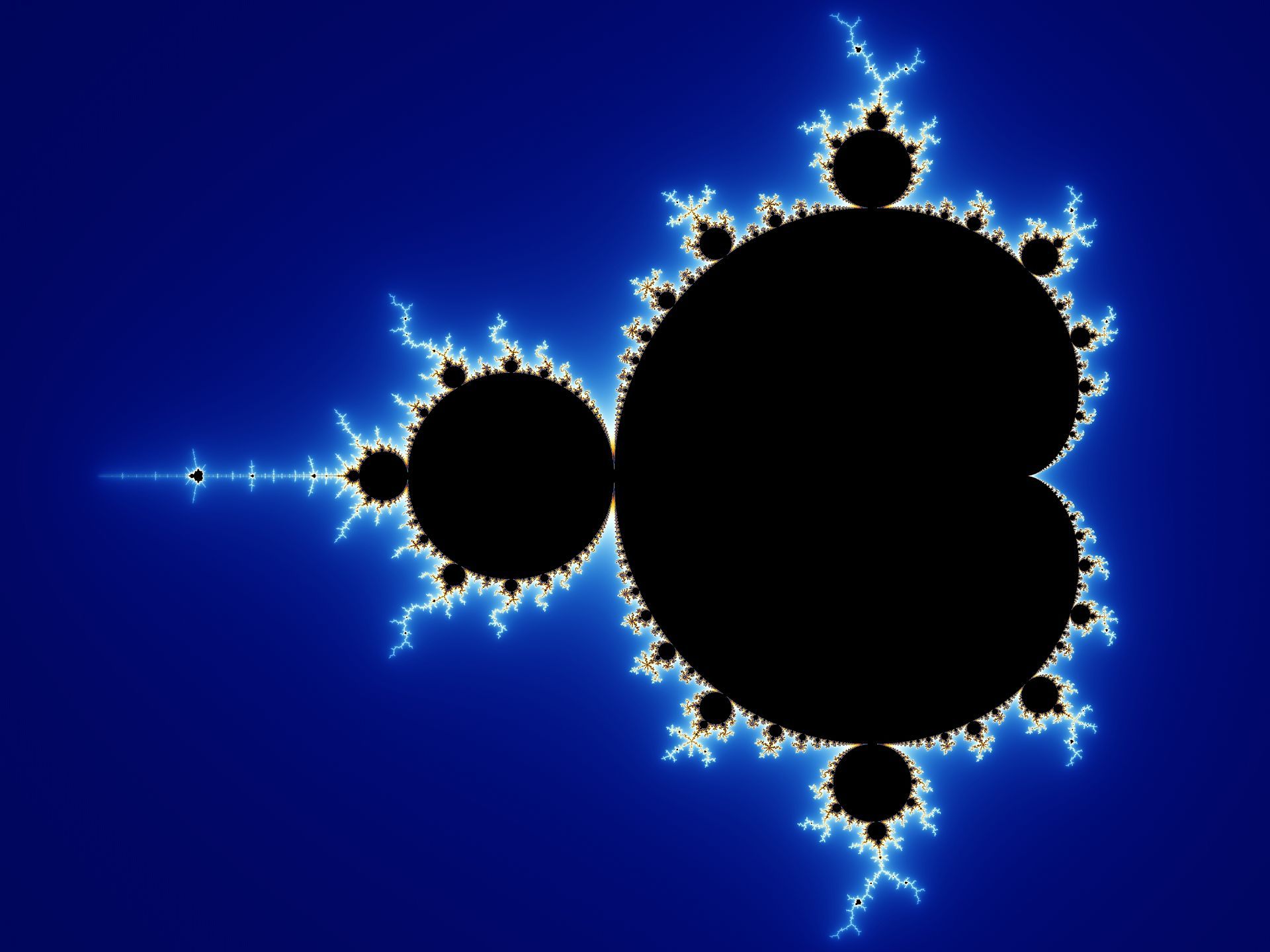
Considered abstractly, the coastline paradox is an awe-inspiring thing, proof of the impossibility of pinning everything down, and of the essential irreducibility of our world. On a practical level, though, it’s a huge pain, says Branden Rishel, a cartographer who works on shoreline restoration in Puget Sound. Like much of Washington State, Puget Sound is full of fjords, endless nooks and crannies carved into the land by glaciers 15,000 years ago. Fjords add a lot of crazy to a coastline—the endlessly crinkly Norway, for example, has about 18,000 miles of coastline with fjords, and a mere 1573 miles if you leave them out.

On top of the pure mathematical strangeness, coasts are constantly changing, says Rishel. Bluffs erode, sea levels rise, land masses slowly rebound from where the glaciers pushed them. Every day, the tides go in, shifting the waterline ten feet, and then back out again. “Beaches change shape with every wave,” Rishel says. “How can you pin that down?”

You can’t—even when you really want to. In 2006, the Congressional Research Service published a memo called “U.S. International Borders: Brief Facts,” meant to ensure everyone was on the same page about exactly how much border we need to think about securing. But once again, there wasn’t even a same page to be on. “The ‘general coastline’ data in this report are based on large scale nautical charts, resulting in a coastline measure for the 50 states totaling 12,383 miles,” the report reads. “Another measure using smaller scale nautical charts more than doubles this measurement to 29,093 miles… while the figures used by the NOAA in administering the Coastal Zone Management program come to 88,612 miles (not including the Great Lakes).”

Sometimes, though, people have to find a way to agree. In Washington State, experts like Rishel use what’s called the “ShoreZone Shoreline,” which was drawn by the Washington Department of Natural Resources in the late 1990s, based on photos and videos taken from low-flying helicopters. The ShoreZone version of the Puget Sound coastline is about 2,500 miles long, Rishel says—a manageable number, or at least better than “infinity.” By agreeing on this approximation, people who work to restore beaches, track where fish spawn, or build waterfront homes can make sure they’re speaking the same geographical language.

But attempted standardization has its own flaws. The ShoreZone shoreline treats some manmade structures, like certain jetties, as though they’re part of the coastline, and leaves others out. “Sometimes the ShoreZone shoreline is dozens of feet from where any respectable shoreline should be,” Rishel says. “In one place it’s 800 feet off and there’s an airstrip in what should be water.” It even managed to miss an entire island.

Rishel expects ShoreZone to be updated soon with LiDAR data, which replaces cameras and human eyes with more precise laser measurements, taken from planes. Still, it’s not going to be perfect. “Over the month or two it took to fly, even the best LiDAR would capture a blurred snapshot of the Sound,” he says. “Winter beach profiles are drastically different from summer ones. Bluffs keep eroding. Landslides happen.”

That’s what happens when we try to work with something that’s literally infinite—we just have to do the best we can.