The Artful Precision of the Creator of ‘Harold and the Purple Crayon’

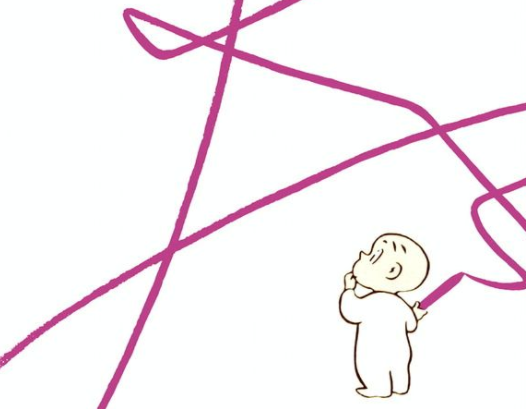
The 1959 children’s novel Harold and the Purple Crayon is a beloved classic, and for good reason. The book is a triumphant celebration of the power of creation and imagination. Crockett Johnson (real name, David Johnson Leisk), the cartoonist and author behind Harold, brought his protagonist’s journey to life with a simple, minimalist drawing style that suggests a child might have made them. Of course, that couldn’t be further from the truth.

“They may look casual, or uncomplicated, but that is actually the result of very careful planning,” says the Johnson biographer Philip Nel, who runs an exhaustive website on the artist’s life and work. In fact, Johnson was a devotee of meticulous precision. In the final decade of his life, he even produced a series of paintings inspired by the laws of geometry and mathematics. The mathematical paintings of Crockett Johnson are far less well known than his famous children’s books, but no less worthy of appreciation.

That Johnson would spend his final years exploring a fine art approach to math is perhaps less surprising when looking back at the peculiar ways he integrated mathematics into his early work. It began in the 1930s, when he was drawing comics for the political publication New Masses, and later, Collier’s. His first major success came in 1942 with the creation of his comic strip, Barnaby, about a young boy (who bore a striking resemblance to the later character of Harold), and his fairy godfather. Johnson wrote and illustrated Barnaby daily until 1946, his preoccupation with precision and mathematics showing through even in this early phase of his career.

One of the most distinct features of the Barnaby comics was their typeset speech balloons. “The normal way you do a speech balloon is that you write text and then you draw the balloon around it, because you don’t know how much space the text is going to take up,” says Nel. But according to Nel, Johnson approached speech balloons the other way around. “[He] drew the balloons first and then figured out in his head about how much space he would need in the balloons, and then had them typeset,” says Nel. “Not many people could do that.”

Then there was Atlas the Mental Genius, a Barnaby character. This odd guest star had a unique habit of speaking in complex algebraic equations. In the beginning, these equations were numerical nonsense, but in later printings of the strips, Johnson went back and replaced them with actual math that could be solved to “say” something. While Barnaby was a fairly sophisticated strip, the inclusion of college-level math was still an odd choice. “Mathematicians thought it was genius, but only mathematicians thought it was genius, because they were the only ones who got the joke,” says Nel.

Johnson eventually moved on to the children’s books he’s best known for today, in particular the Harold series. He wrote and illustrated seven Harold titles between 1955 and 1963, all revolving around a young boy who draws worlds for himself with his magic crayon. Johnson brought his analytical eye to these charming books as well. “Each book is in fact one giant drawing that Johnson figured out in advance, and then had Harold draw, revealing his progress a page at a time. Harold does not erase, and only rarely crosses out,” says Nel.

It wasn’t until after the release of his final Harold book, Harold’s ABC’s, that Johnson began working on the final great works of his life, his mathematical paintings. “He starts on those in 1965,” says Nel. “At that point, he has had a full career as a comic book artist and as a children’s book writer and artist. And the mathematical paintings are the third phase of his career.”

Working from a 1956 textbook called The World of Mathematics, Johnson took what had been implied in much of his earlier work and made it literal, painting equations and proofs as colorful geometric expressions of their math. For instance, in one of his early mathematical pieces, Proof of the Pythagorean Theorem (Euclid), precise triangles and shapes act as a visual breakdown of the famed mathematician’s proof. For comparison, see below:

Relatively new to the world of fine art, Johnson created his paintings using rudimentary materials he could pick up at the hardware store. Instead of canvas, he painted on masonite boards. “He found canvas intimidating,” says Nel. Johnson even had his colors mixed at the store rather than doing it himself. “He was literally using house paint for these.”

Despite his lack of a formal advanced mathematical education, Johnson was fascinated by complex algebra. Eventually, he began experimenting with his own mathematical theorems. “He would paint versions of a problem until he arrived at a solution, and when he arrived at a solution, he would correspond with mathematicians to try and get the algebra,” says Nel.

Through his own experimentation, combining his artistic experience with his passion for math, Johnson was eventually able to publish two completely original mathematical proofs in scholarly journals. One, titled “A Construction for a Regular Heptagon,” was published in a 1975 edition of the Mathematical Gazette, providing an alternative to a proof originally credited to Archimedes.

Despite their clear skill and innovation, Johnson’s own attitude toward his mathematical works was always conflicted, as he never seemed to get comfortable enough to consider himself a fine artist. With the help of an art world friend, he was able to stage a couple of gallery shows, and according to Nel, he even sent a print to the Museum of Modern Art in New York, but they weren’t interested in showing his work. Nel tells of a particular instance when a friend told Johnson that one of his paintings could sell for at least $10,000. “Johnson responded with a scornful look, saying, ‘$10,000? No! If I sold one, then it would give the others value. And if the others have value, then on my death bed, I would impoverish my heirs.’ This was a joke, because he had no children, and therefore no heirs to pay taxes on an inheritance.” Nel says that Johnson often used humor to hide his artistic insecurities.

Johnson continued to produce new mathematical paintings until his death in 1975, creating over 100 such works. He thought of them all as one cohesive piece, best understood as a single body of work, and whether out of a lack of confidence in the pieces or a true desire not to commercialize them, he never sold them. “When he died, the work was given to the Smithsonian with the understanding that they would keep it together, and not sell it,” says Nel.

Johnson is still chiefly remembered for Harold and his purple crayon, but the Smithsonian’s National Museum of American History still holds 80 of Johnson’s mathematical paintings in its collection, preserving the lesser-known legacy of the artist’s precise imagination.